vnhas.blogg.se - Results become more accurate standard deviation

RESULTS BECOME MORE ACCURATE STANDARD DEVIATION HOW TO
RESULTS BECOME MORE ACCURATE STANDARD DEVIATION FULL

RESULTS BECOME MORE ACCURATE STANDARD DEVIATION FULL

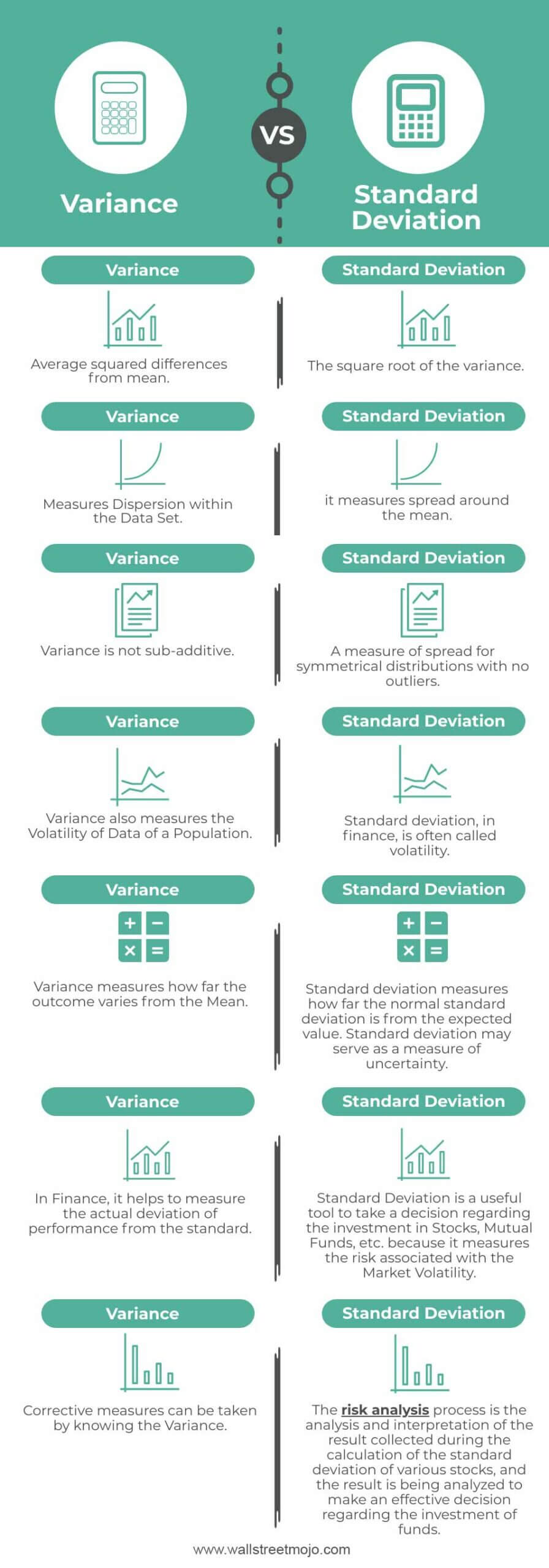
Just like the sample mean, a sample standard deviation exists for samples of a population, if you are not given data or a probability distribution for the full population. Standard deviation will appear again in year \(12\) when looking at continuous distributions, so make sure you’re comfortable with the concept! \(Standard \ deviation=σ=\sqrt(Variance)\) In general, you can obtain the standard deviation by taking the square root of the variance, even if you are dealing with probability distributions instead of data sets: The standard deviation scales the same way as our data, making it a useful statistic to measure. understand that a sample mean, \(\overline\)Īnd our old variance was \(1.6\), which means our old standard deviation was \(\sqrt1.6\) which is half of our standard deviation for our doubled second set.recognise the variance, \(Var(?)\), and standard deviation \((?)\) of a discrete random variable as measures of spread, and evaluate them in simple cases (ACMMM141).use discrete random variables and associated probabilities to solve practical problems (ACMMM142) AAM.NESA requires students to demonstrate proficiency in the following syllabus dot points:

Sample vs population standard deviation.You will encounter the standard deviation again when considering probability distributions in year 12.Īdditionally, a good understanding of standard deviation is key for using statistics appropriately after high school. Variance and standard deviation are measures of spread, extending upon your statistics knowledge from earlier years. Year 11 Advanced Mathematics: Statistics for Variance and Standard Deviation You’re not the only one! In this blog post, we’ll cover the meaning of Variance and Standard deviation to help you prepare for Year 11 Advanced Maths.

RESULTS BECOME MORE ACCURATE STANDARD DEVIATION HOW TO

Besides, this way the cost of surveying the entire population.Are you confused about how to calculate and manipulate the variance and standard deviation for related sets of data?

Also this process is easy to estimate as we don’t have to cover the entire size of the things rather we just have to focus on the small sample part of the entire thing and this small sample represents the entire thing.

And as the heading the static are never accurate use of margin of error provide better resolution to the estimated result from any polls or survey.

It is a very useful method for estimating any value in this method like Calculations assuming random sampling, Different confidence levels plays an important role and also effect of sample size matters a lot.

Statistics aren’t accurate Use of margin of Error Using the formula of the margin of error find the value.Ĭalculate the percentage of the step 3 and then we get thee value of the margin of error is 2.52%. A 90% confidence interval has a z-score of 1.64. you’ll need to reference this critical values. Calculate Z-ScoreĬalculate the z-score that goes with confidence interval. It means that they answered according to the statement in this example 38% scientist responded positively. of people who agreed climate change was not caused by human pollution. Find the MoE for a 90% confidence interval Calculate P-hatĬalculate P-hat(sample proportion) by dividing the no. To calculator proportion we need sample proportion, sample size and z-scoreĪ group of people did a survey on 1000 scientists and 380 of thought that climate change was not caused by human pollution.